Tags
Arthur C Clark, cosmos, fractal geometry, fractal pattern, fractals, Fractals: The Colors of Infinity, Mandelbrot Set, Michael Barnsley, Physics Today, Professor Benoît Mandelbrot, Professor Ian Stewart, Scientific Illustration for the Research Scientist, Steven Hawking
.
How is it that there are infinities?
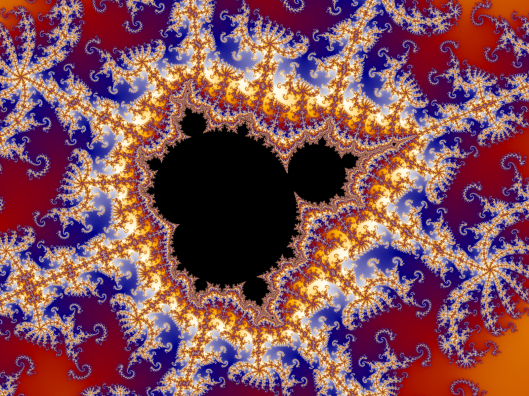
Since discovery of fractal geometry, infinities are everywhere. The term “fractal” was first used by mathematician Benoît Mandelbrot in 1975. A fractal is a natural phenomenon or a mathematical set that exhibits a repeating pattern that displays at every scale. If the replication is exactly the same at every scale, it is called a self-similar pattern. As mathematical equations, fractals are usually nowhere differentiable. The mathematical roots of the idea of fractals have been traced throughout the years as a formal path of published works, starting in the 17th century with notions of recursion. The word comes fro the Latin frāctus meaning “broken” or “fractured” and fractals are not limited to geometric patterns, but can also describe processes in time. Fractal patterns are found in structures and sounds, in nature, and technology.
.
“The most beautiful thing we can experience is the mysterious. It is the source of all true art and science.”
~ Albert Einstein
.
Fractals: The Colors of Infinity
Arthur C. Clark
Video Description
Arthur C. Clarke presents this unusual documentary on the mathematical discovery of the Mandelbrot Set (M-Set) in the visually spectacular world of fractal geometry.
This show relates the science of the M-Set to nature in a way that seems to identify the hand of God in the design of the universe itself. Dr. Mandelbrot in 1980 discovered the infinitely complex geometrical shape called the Mandelbrot Set using a very simple equation with computers and graphics.
The Mandelbrot set – someone has called it the thumb-print of God – is one of the most beautiful and remarkable discoveries in the entire history of mathematics.
With Arthur C. Clarke as narrator and interviews with a number of notable mathematicians, including Benoît Mandelbrot, this program graphically illustrates how simple formulas can lead to complicated results: it explains the set, what it means, its internal consistency, and the revolutions in thought resulting from its discovery. Asked if the real universe goes on forever, Stephen Hawking defines its limit of smallness; the Mandelbrot set, on the other hand, may go on forever.
The invention of the silicon chip in the 1970′s created a revolution in computers and communication and hence transformed our way of life. We are now seeing another revolution which is going to change our view of the universe and give us a better understanding of its’ working.
The film explores the fractal universe helped by: Professor Ian Stewart of the Mathematics Institute, University of Warwick, an author of over 100 published scientific works; Dr. Michael Barnsley, former professor of mathematics at Georgia Institute of Technology who received a 2.5 million dollar government grant in 1991 to develop a fractal image compression systems.
.
Fractals: The Colors of Infinity
A 1995 Television Movie
The film celebrates the discovery of the Mandelbrot Set – one of the most profound and remarkable events in the history of mathematics. The programme explores the revolutionary world of Fractal Geometry – its far-reaching and often unexpected implications – its powerful and revolutionary applications.
Director: Nigel Lesmoir-Gordon
Writers: Arthur C. Clarke, Nigel Lesmoir-Gordon
.
Thanks for visiting.
 Eric
Eric
.
see also
Our Fractal Nature
Fractal patterns and geometry
New physics – spinning
What is quantum touch?
Is consciousness physics?
A geometric solution for gravity – new physics spinning
Strong force – electromagnetic fluctuations
.
.
Here is a glitch that every banking executive probably must know about:
.
.
.


Interesting.